Motivation

Bright matter-wave solitons are small dispersionless wave packets that keep its shape while propagating. Typically, this shape-preserving propagation is the result of a delicate balance between attractive interaction energy and dispersive kinetic energy. (Have a look at our project with Floquet solitons for bright matter-wave solitons with repulsive interactions.) The balancing condition relates parameters of the soliton, such as size, atom number, and interaction strength. It is easy to destroy this balance by changing the soliton size. For example, making the soliton larger decreases the kinetic energy while making the soliton smaller increases it.

What happens if we change the size of the soliton, e.g. make it slightly too large? Does the soliton return to its favorite size or does it fall apart?

The answer is: both. The soliton starts to oscillate for small size changes - getting periodically larger and smaller. Its oscillation frequency is a bit unusual, because there is no trap or external potential that could set it. Instead, the frequency is a property just of the soliton, depending on parameters such as atom number, interaction strength, and size.

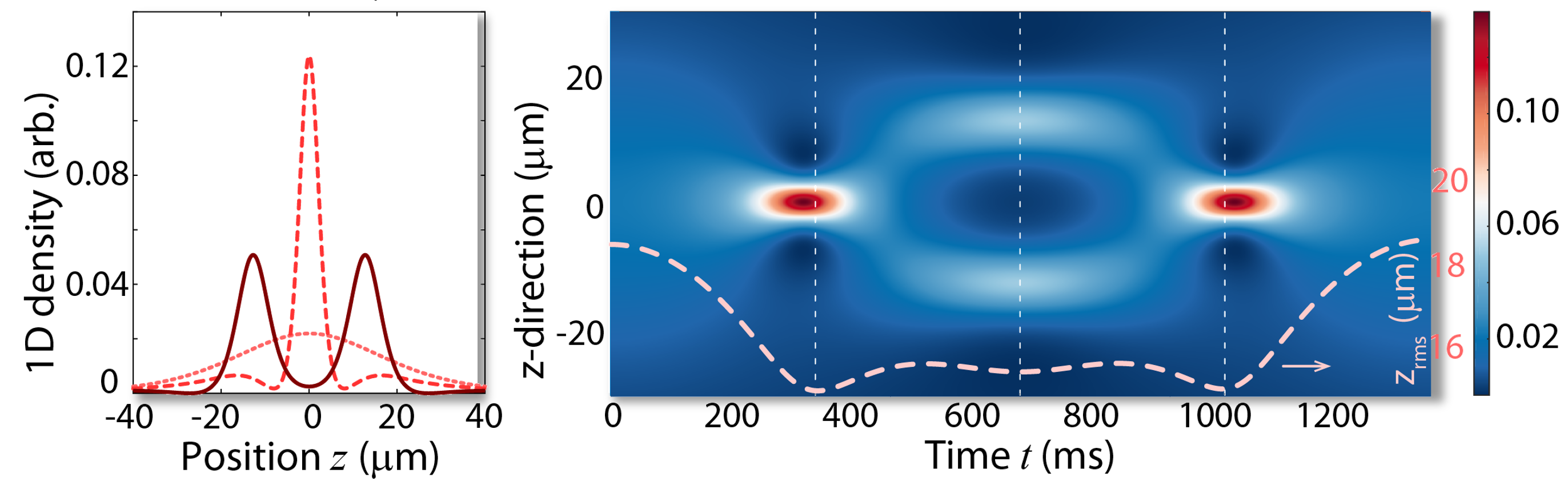
There are two more options for the soliton to react when its size is far too large: (1) The soliton can shed atoms to get smaller. For example, when it is too large, it can eject atoms until it regains an atom number that fits to its larger size. (2) The soliton starts to oscillate but with a strange breathing-like motion, creating multiple sub-peaks during the oscillation (see cover image at the top of the page). Those oscillations are called higher-order oscillations and appear only for very specific sizes and with discrete frequencies.

Project summary

We experimentally studied the excitation modes of bright matter-wave solitons in a quasi-one-dimensional geometry. The solitons were created by quenching the interactions of a Bose-Einstein condensate of cesium atoms from repulsive to attractive in combination with a rapid reduction of the longitudinal confinement. A deliberate mismatch of quench parameters allowed us to excite breathing modes of the emerging soliton and to determine its breathing frequency as a function of atom number and confinement. In addition, we observed signatures of higher-order solitons and the splitting of the wave packet after the quench [Phys. Rev. Lett. 123, 123602 (2019)].